Many students studying higher mathematics in their senior years probably wondered: where are differential equations (DE) used in practice? As a rule, this issue is not discussed in lectures, and teachers immediately move on to solving differential equations, without explaining to students the application of differential equations in real life. We will try to fill this gap.
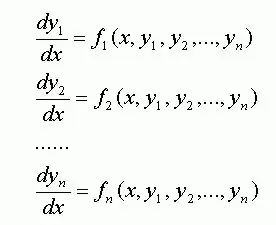
Let's start by defining a differential equation. So, a differential equation is an equation that connects the value of the derivative of a function with the function itself, the values of the independent variable and some numbers (parameters).
The most common area in which differential equations are applied is the mathematical description of natural phenomena. They are also used in solving problems where it is impossible to establish a direct relationship between some values describing a process. Such problems arise in biology, physics, economics.
In biology:
The first meaningful mathematical model describing biological communities was the Lotka - Volterra model. It describes a population of two interacting species. The first of them, called predators, in the absence of the second, dies out according to the law x ′ = -ax (a> 0), and the second - prey - in the absence of predators multiplies indefinitely in accordance with the law of Malthus. The interaction of these two types is modeled as follows. Victims die out at a rate equal to the number of encounters of predators and prey, which in this model is assumed to be proportional to the size of both populations, i.e., equal to dxy (d> 0). Therefore, y ′ = by - dxy. Predators reproduce at a rate proportional to the number of prey eaten: x ′ = -ax + cxy (c> 0). System of equations
x ′ = -ax + cxy, (1)
y ′ = by - dxy, (2)
a predator-prey describing such a population is called the Lotka-Volterra system (or model).
In physics:
Newton's second law can be written in the form of a differential equation
m ((d ^ 2) x) / (dt ^ 2) = F (x, t), where m is the mass of the body, x is its coordinate, F (x, t) is the force acting on the body with coordinate x at time t. Its solution is the trajectory of the body under the action of the specified force.
In economics:
Model of natural growth of output
We will assume that some products are sold at a fixed price P. Let Q (t) denote the amount of products sold at time t; then at this point in time the income is equal to PQ (t). Let a part of the specified income be spent on investments in the production of products sold, i.e.
I (t) = mPQ (t), (1)
where m is the investment rate - a constant number, and 0






