Aristarchus of Samos is an ancient Greek astronomer, philosopher of the 3rd century BC. He was the first to propose a heliocentric system of the world, developed a scientific method for determining the distance to the Sun and Moon, their sizes.

There is very little information about the life of the ancient Greek mathematician and astronomer. It is known that he was born on the island of Samos. Nothing is known about the years of his life. Usually they indicate data based on indirect information: 310 BC. e. - 230 BC e. Nothing is known about the personal life of the scientist, his family.
Founder of heliocentrism
According to Ptolemy, in 280 BC. Aristarchus watched the solstice. This is practically the only authoritative date in the biography of the scientist. The astronomer was a student of the great philosopher Straton of Lampascus. For a long time, according to the assumptions of historians, the astronomer worked at the Hellenistic scientific center in Alexandria.
The scientist was accused of atheism after his statement about the heliocentric system. The consequences of this accusation are unknown. In one of the works of Archimedes, there is a mention of the astronomical system of Aristarchus, described in detail in the unpreserved work of the astronomer.
He believed that the movements of all planets occur inside the fixed sphere of static stars. The sun is located in its center. The Earth moves in a circle. Aristarchus' constructions became the highest achievements of the heliocentric hypothesis. Because of the author's courage, he was charged with apostasy. The scientist was forced to leave Athens. In the original, the work of the astronomer "On the distances and sizes of the Moon and the Sun" was published in Oxford in 1688.
The name of Samos is always mentioned when studying the history of the development of views on the structure of the universe and the place of the Earth in it. Aristarchus of Samos was of the opinion about the spherical structure of the universe. Unlike Aristotle, the Earth was not the center of universal circular motion for him. It took place around the sun.
Scientific method for calculating distances between celestial bodies
The ancient Greek scientist came closest to the real picture of the universe. However, the proposed design did not gain popularity at that time.
Heliocentrism believes that the Sun is the central celestial body. All planets revolve around it. This view is the opposite of geocentric construction. The point of view put forward by Aristarchus of Samos was understood by the fifteenth century. The Earth revolves around its axis in one sidereal day, and around the Sun - in a year.
The result of the first movement is the apparent reversal of the celestial sphere, the second - the annual movement of the star among the stars along the ecliptic. The Sun is considered stationary relative to the stars. According to geocentrism, the Earth is at the center of the Universe. This theory has dominated for centuries. It was not until the sixteenth century that the heliocentric doctrine began to gain prominence. Aristarchus' hypothesis was recognized by the Copernicans Galileo and Kepler.
In the essay of the scientist "On the distances and magnitudes of the Moon and the Sun" calculations of distances to celestial bodies, attempts to indicate their parameters are shown. Ancient Greek scholars have spoken out on these topics many times. According to Anaxagoras of Clazomea, the Sun is much larger than the Peloponnese. But he did not provide a scientific basis for the observation. There were no calculations of the distances to the stars, there were no observations by astronomers. The data was just made up.
However, Aristarchus of Samos used a scientific method based on observations of eclipses of luminaries and lunar phases.
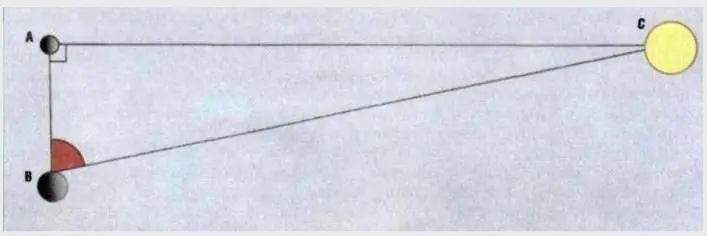
Explanations of the method
All formulations were based on the hypothesis that the Moon reflects the light of the Sun, has the shape of a ball. From this, the statement followed: when the Moon was placed in square, when it was cut in half, the angle of the Sun - Moon - Earth is right. With the available data on the angles and the "solution" of the right-angled triangle, the ratio of the distances from the Moon to the Earth is established.
Aristarchus's measurements show that the angle is 87 degrees. The result gives information that the Sun is nineteen times more distant than the Moon. Trigonometric functions were unknown at that time. To calculate the distances, the scientist used very complex calculations. They are described in detail in his essay. The following is information about solar eclipses. The researcher was well aware of what they happen when the moon obscures the star. For this reason, the astronomer pointed out that the angular parameters of celestial bodies are approximately the same. The conclusion was the assertion that the Sun is as many times larger than the Moon, as far as it is. That is, the ratio of the radii of the stars is approximately equal to twenty.
This was followed by attempts to determine the size of the stars in relation to the Earth. Analysis of lunar eclipses was used. Aristarchus knew that they occur when the moon is in the cone of the earth's shadow. He determined that in the region of the Moon's orbit, the cone is twice as wide as its diameter. The famous astronomer made a conclusion about the ratio of the radii of the Sun and the Earth. He gave an estimate of the lunar radius, claiming that it is three times smaller than the earth's. This is practically equal to modern data.
The distance to the Sun was underestimated by ancient Greek scientists by about two dozen times. The method turned out to be rather imperfect and prone to errors. However, it was the only one available at the time. Aristarchus did not calculate the distances to the day and night stars, although with knowledge of their angular and linear parameters, he could do this.
The work of the scientist has great historical significance. She became the motive for studying the third coordinate. As a result, the scales of the Universe, the Milky Way, the Solar System were revealed.

Improving the calendar
The great man also influenced the improvement of the calendar. This became another facet of his work. Aristarchus established the length of the year at 365 days. This is confirmed by the writer Censorion. The astronomer proposed the use of a calendar period of 2434. This interval was several times larger than the period of 4868 years, the "Great Year of Aristarchus" and was a derivative.
The Vatican Chronicles consider the ancient Greek scientist to be the first astronomer to create different meanings for the length of the year. Sidereal and tropical values are not equal due to the precession of the planet's axis. If the Vatican lists are correct, then these differences were first identified by the ancient Greek scholar, who is the discoverer of the precession.
It is known that the great astronomer of antiquity created trigonometry. According to Vitruvius, he improved the sundial, invented their flat version.
Aristarchus also studied optics. He assumed that when light falls on objects, their color appears, and colors are not distinguishable in the dark. There are suggestions that he set up experiments to determine the resolving sensitivity of the eye. Contemporaries recognized the scientific contribution of Aristarchus. He is forever included in the list of the greatest mathematicians of the planet.
His work was included in the manuals compulsory for beginning ancient Greek astronomers, the works were cited by Archimedes.

In honor of the ancient Greek scientist, they received the names of an asteroid, a crater on the Moon, and an air hub on the island of Samos.






